Tip 1: Card frequency in cribbage
Tip 2: Leading a 5
Tip 3: Defending against a two-on-one
Cribbage is played with a standard 52-card deck, divided into four suits and thirteen ranks. Although suit enters the picture when a player gets a flush or His Nobs, it's rank that's of primary importance. Not all ranks are created equal. Because face cards have a pip value of ten, and because we award two points for a fifteen combination, 5s are, a priori, the most valuable cards in the deck (making cribbage perhaps the world's only card game where this is the case). Points are also scored for runs, and since these are not allowed to cycle around from a K to an A, the usefulness of edge cards (A, 2, Q and K) is correspondingly reduced.
Since different card ranks tend to have different values, it stands to reason that certain ranks are more likely to be retained after discarding. We can calculate that any particular rank has a 39.7% chance of appearing among your opponent's initial six cards, but do we know how likely is it to appear among her final four? Well, thanks to some work by a few enterprising researchers, we do.
The measure in question is card frequency by rank, which is expressed as the percentage likelihood that, on a random deal, your opponent will be holding at least one card of the specified rank after the toss. Obviously these numbers are linked to discarding decisions, and thus will be different for pone and dealer.
I know of three good sources for card frequency data. All are encapsulated in the following table. The figures are for normal, non-endgame situations.
| Rank | Pone | ||
| Hessel | Schempp | Ras | |
| A | 21.5% | 20.9% | 19.9% |
| 2 | 25.4% | 25.0% | 25.2% |
| 3 | 28.1% | 27.8% | 27.0% |
| 4 | 28.7% | 28.7% | 29.5% |
| 5 | 38.5% | 38.6% | 39.0% |
| 6 | 28.3% | 28.7% | 28.2% |
| 7 | 25.6% | 25.1% | 25.8% |
| 8 | 25.5% | 25.2% | 26.0% |
| 9 | 25.0% | 25.2% | 25.6% |
| 10 | 23.5% | 23.2% | 22.8% |
| J | 29.6% | 30.0% | 28.4% |
| Q | 19.8% | 19.0% | 20.3% |
| K | 13.4% | 12.3% | 14.7% |
| Rank | Dealer | ||
| Hessel | Schempp | Ras | |
| A | 25.6% | 25.6% | 25.0% |
| 2 | 24.0% | 23.8% | 23.9% |
| 3 | 24.2% | 24.1% | 25.1% |
| 4 | 27.9% | 28.0% | 28.4% |
| 5 | 28.2% | 28.5% | 28.7% |
| 6 | 26.1% | 25.6% | 25.6% |
| 7 | 22.5% | 21.1% | 21.8% |
| 8 | 22.8% | 21.7% | 22.7% |
| 9 | 25.7% | 25.3% | 25.0% |
| 10 | 29.1% | 29.0% | 29.1% |
| J | 26.9% | 27.3% | 27.3% |
| Q | 26.9% | 27.5% | 27.0% |
| K | 27.0% | 26.9% | 26.7% |
Like discarding averages, these figures are standardized results drawn from thousands of samples and calculations. While they are useful, it is important to remember that they are an abstraction. In a real game the likelihood of your opponent having a particular rank will depend on the starter and the six cards you were dealt, since she obviously cannot have any of these. And naturally, once she starts playing her cards, you will get a more concrete idea of her hand composition.
The three sets of statistics were generated using three distinct methodologies. Craig Hessel's figures come from the same calculation routine he developed to compile his table of discard averages (see my articles Discarding to your crib and Discarding to opponent's crib). Hessel's original routine disregarded suit entirely, and since the possibility of retaining a flush was thereby removed, the incidence of pairs was somewhat overstated. He has since revised his algorithm to correct this, and it is the newer and more accurate results that I cite above.
Tim Schempp's percentages are derived from the hand frequency data included with his table of pegging averages. The source data were sampled from computer simulations of non-endgame discarding situations. Since Schempp's discarding algorithm ignores suit, the incidence of pairs is slightly exaggerated. This explains why his totals for each rank are, on average, a trifle lower than Hessel's.
George "Ras" Rasmussen's statistics come from the discard data he gathered from his own over-the-board games between 1990 and 1998. It is the same data set used to generate his discard averages. Although Ras did not specifically track card frequency in four-card hands, he did note the exact incidence of each of the 91 possible discards in his sample set, making it possible to extrapolate card frequency numbers by assuming a uniform distribution of ranks among the original six-card hands. Like the other numbers, Rasmussen's pertain to non-endgame situations. His methodology was to throw out data for cribs that were not actually counted in the game (i.e., because one player had already won).
Although they were gathered using very different techniques – empirical calculation, computer simulation, sampling from expert games – these three sets of numbers agree very closely. Just how closely can be seen by graphing the results, which also makes them easier to visualize.
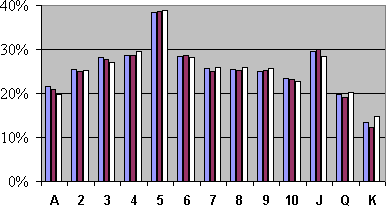

None of the results for any rank vary by more than 2.4% between sources. The majority are within 1%. As a further check on their reliability, I compared these statistics to those generated from a log file of 500 games I played against Cribbage for Windows 97. I found that my results correlated extremely closely with those of Hessel, Schempp and Rasmussen. We can be confident that these two graphs are an accurate representation of card frequency in cribbage hands.
Let's take a closer look at the numbers, starting with pone. It's no surprise that the card pone is most likely to hold is a 5. This rank occurs in 38.5% to 39.0% of pone's four-card hands. Comparing that to the 39.7% likelihood of being dealt one or more 5s should give you a idea of how seldom this card gets passed to an opponent's crib. The second most frequently held card is the J, which is obviously preferred over other ten-cards because of the possibility of His Nobs.
Pone will hold onto mid-cards with average frequency. There is a slight bump on 4s and 6s, probably because these cards are often held in combination with a 5. A more significant feature is the drop-off in edge cards. Whereas Js appear in about 30% of pone hands, Qs appear in only 20%, and Ks are present in only 13%. There is a similar, though less dramatic, drop-off with 3s, 2s and As, all of which appear less often than 4s, with progressively decreasing frequency. Although they are valued as pegging cards, As and 2s just don't factor into enough runs to maintain parity with their higher neighbors.
What are the implications for practical play? Well, the conventional wisdom that 5s and Js are the easiest cards to trap when pegging against pone is clearly upheld. If you're dealer, saving combinations like 3-4, 4-6 and 6-7 for last can be remarkably effective – pone will often have a lone 5 lurking behind a trio of low and/or mid-cards. Likewise the well-known trick of retaining a pair of Js for last in hopes of tripling a lone J is a valid tactic against pone. But trying the same ploy with a pair of 10s, Qs or Ks is much less likely to succeed.
If you're trying to hold down pone's pegging, then the percentages tell you he's most likely to be able to pair a 5, and least likely to be able to pair a K. It's important to keep this in perspective though. If you're defending with 4-5-10-K and pone leads a 3, your safest reply is ordinarily the K. But if the starter is a 10, then your safest reply is the 10, since the duplication of this rank by the starter is more significant than pone's general preference for 10s over Ks. In general, a matched card is statistically safer than an unmatched card, but 5s and Js are exceptions. A duplicated 5 is no safer against pone than a lone A, 2, 3 or any other card besides a J. And a duplicated J is more likely to be paired by pone than a lone K, and about equally likely to be paired as a lone Q. So if you're holding J-J-Q-K as dealer, your safest reply to an A through 10 lead is your K, not one of your Js.
Take another look at pone's Q/K drop-off. If your opponents aren't hanging onto these cards, then where are they going? Into your crib, of course. Now you know why certain wide card combinations return a bit more in your crib when they contain a Q or K instead of a 10.
| Toss: | Average in your crib: | |||||
| Consensus | Hessel | Bowman | Ras | |||
| A-10 | 3.43 | 3.37 | 3.42 | 3.51 | ||
| A-Q | 3.43 | 3.39 | 3.40 | 3.50 | ||
| A-K | 3.41 | 3.42 | 3.44 | 3.36 | ||
| 2-10 | 3.57 | 3.51 | 3.50 | 3.71 | ||
| 2-Q | 3.62 | 3.52 | 3.49 | 3.86 | ||
| 2-K | 3.54 | 3.55 | 3.49 | 3.57 | ||
| 3-10 | 3.56 | 3.61 | 3.56 | 3.51 | ||
| 3-Q | 3.61 | 3.62 | 3.55 | 3.65 | ||
| 3-K | 3.70 | 3.66 | 3.55 | 3.89 | ||
| 4-10 | 3.59 | 3.62 | 3.56 | 3.60 | ||
| 4-Q | 3.60 | 3.63 | 3.55 | 3.63 | ||
| 4-K | 3.61 | 3.67 | 3.55 | 3.61 | ||
| 5-10 | 6.66 | 6.68 | 6.61 | 6.70 | ||
| 5-Q | 6.63 | 6.71 | 6.59 | 6.59 | ||
| 5-K | 6.67 | 6.70 | 6.59 | 6.73 | ||
| 6-10 | 3.20 | 3.15 | 3.13 | 3.31 | ||
| 6-Q | 3.31 | 3.08 | 3.12 | 3.73 | ||
| 6-K | 3.15 | 3.13 | 3.12 | 3.21 | ||
| 7-10 | 3.28 | 3.10 | 3.14 | 3.59 | ||
| 7-Q | 3.25 | 3.17 | 3.19 | 3.39 | ||
| 7-K | 3.29 | 3.21 | 3.19 | 3.47 | ||
| 8-Q | 3.18 | 3.16 | 3.20 | 3.19 | ||
| 8-K | 3.15 | 3.20 | 3.21 | 3.04 | ||
| 9-Q | 3.00 | 2.97 | 3.03 | 2.99 | ||
| 9-K | 3.07 | 3.05 | 3.08 | 3.07 | ||
With pone, the difference in frequency between the most and least favorite rank is over 25%. With dealer, the corresponding spread is less than 8%. Since dealer's most valuable cards can find themselves in the hand or crib, the distribution of ranks in dealer's four-card hands is much more flat.
The edge card drop-off we saw for pone is completely absent for dealer. An A gets held a trifle more often than a 2 or 3. And Js, Qs and Ks are each held with equal frequency. There are slight bumps on 4s and 6 (which are often held with a 5), and a notable dip on 7s and 8s. The latter feature results from dealer's tendency to toss mid-cards from mixed hands like the following:
A-A-8-8-Q-Q
A-3-6-7-Q-K
2-3-7-8-10-J
Knowing about this mid-card dip can influence how you play your cards as pone. Suppose you're holding the following:
7-8-J-J
You have a choice between leading a J and leading one of the mid-cards. Knowing that dealer is statistically less likely to be holding a 7 or 8 than a 5 or J, you decide to lead the 8. Good choice, but not just because of card frequency. It also helps you stay out of trouble if dealer does score on your lead. If he takes a 15-2 with a 7, you can either pair him or else break with a J, leaving you with the flexible 7-J. But if he scores a 15-2 off a J lead instead, you're then stuck playing your second J, since you cannot safely play your 7 or 8. This gives up a 31-2 if dealer has a 6 – not too unlikely if he has a 5 ‐ and leaves you with touching cards, which are more likely to get trapped into a run later.
The 7/8 dip also helps explain why mid-cards often seem more productive in your opponent's crib than in your own. If you've studied discarding averages for dealer and pone, you may have noticed that while most two-card combinations are worth roughly one point more to your opponent's crib than they are to your own crib, with mid-card combinations (such as 6-7 and 8-8) the difference is closer to 1¼ points.
Since 7s and 8s are disproportionately absent from dealer's hands, something else must be taking up the slack. That something is 5s and ten-cards, which are slightly more likely to be retained than discarded. This is not surprising when you consider that although there are sixteen low cards (A through 4) and sixteen mid-cards (6 through 9) in the deck, there are a total of twenty 5s and ten-cards. If dealer gets a 5 among her original six cards, it's likely she'll also have a couple of ten-cards, in which case she'll usually keep them all together. If you're pone, it's worth paying particular attention to possible 5 traps, since if dealer does have one of these cards, it'll often be accompanied by ten-cards, leaving the door open for standard traps like these:
6 K 6 5 4 (31-5)
9 K 4 5 3 (31-5)
8 K J (28-1)
K 7 5 6 (28-4)
5 (5-1)
Let's see if we can apply what we know about dealer card frequency to an old conundrum: leading from ten-cards. Say you're holding 5-J-Q-K as pone. For one reason or another, you've ruled out leading the 5. Which card should you lead? The current prevailing opinion is articulated thusly by DeLynn Colvert in Play Winning Cribbage:
"Lead the K. The least likely ten-card held by Jake will be the K. You do not want to entice a pair here."
Colvert specifically advises against leading a lone J, "the most likely ten-card held by Jake". Dan Barlow, in Cribbage for Experts, concurs with the K lead. But a different view is offered by George Rasmussen:
"Although [leading the K] is commonly believed [to be best], and is espoused in DeLynn Colvert's book, it is not so in actual play. The reason is that the J is the key connector among the ten-cards and will be discarded to dealer's crib more than any other ten-card: with the 5, with another J, as a 10-J or J-Q combination. The J will also be discarded with a middle card or a small card. This will nearly always be done in preference to putting the K in the crib. Dealer will often pair your K lead thinking you led it believing it was safest lead of the ten-pointers. Think of how often you discard the J to your own crib, and your rationale for doing so. Once you've done this, it will become readily apparent why the J is the safest ten-card lead you can offer the dealer."
Moreover, Ras states that by leading a single J
"...you're rid of it on the opening play. That danger represented by the trap of a single J is considerable. The J is considered by most to be the second most available card to trap in cribbage. Only the 5 is more frequently trapped."
What to do then? Well, the numbers tell us that dealer is equally likely to have a J, Q or K, so it turns out the conventional wisdom, as rendered by Colvert, is wrong. To be sure, Rasmussen also misstates the case somewhat when he suggests that dealer is less likely to have a J than another ten-card, but his point is still sound. A J is no more vulnerable to being paired than a Q or K, but it is more vulnerable to being trapped into a run, both because it has more neighboring cards, and because dealer is likely to be specifically gunning for it. Therefore the safest plan is to get it out of the way first by making it your opening lead.
A more aggressive ten-card lead would be the K, which saves the J-Q combo for later, thereby maximizing your chances of trapping dealer into a pair or run on the second play series. Leading the Q, advocated by Jarvis and Wergin and formerly my own personal choice, turns out to be inferior. It offers no more safety than leading the J, and because it breaks up your run, it provides less offensive potential than leading the K. It's true that a Q lead prevents dealer from safely playing a 10, something he could do if you led the K. But if dealer is really trapped with four ten-cards, he'll probably just pair your Q lead anyway instead of risking a run or pair with a 10 reply.
Of course, if one of your ten-cards is duplicated by the starter or by a card you tossed, then that card will be the safest lead regardless of its rank.
One other bit of conventional wisdom dispelled by card frequency stats concerns J traps. We've seen that these are very effective against pone, but most authorities, including both Colvert and Rasmussen, will encourage you to try them against dealer as well, usually by saving a pair of Js for last in an attempt to triple a lone J. (With J-J-Q-K, for instance, you would lead the K, hoping to triple dealer's J at the end.) This is certainly a legitimate tactic if you need to peg six points, but there's really no reason to reserve this treatment for Js as opposed to, say, Qs and Ks. In fact, a card you're more likely to be able to triple is a 10, the one ten-card that dealer holds in disproportion to the others (10s are not particularly valuable in dealer's crib, and they'll often be held in combination with mid-cards). If you're holding a pair of 10s as pone, consider leading away from them. You should also think twice before leading a lone 10.
It's worth reiterating that this discussion of card frequency pertains to normal play. In pegging-critical situations, you can count on a very different distribution of opponent's cards, including a preponderance of low cards and magic elevens. Nevertheless, it's worth getting familiar with these numbers. They can tell you things about your opponent's hand that he doesn't want you to know.
- Republished from Cribbage Forum by permission. Text copyright © 2002 by Michael Schell. All rights reserved.
Next